What Best Describes the Orthocenter of a Triangle

0 0 0 0 Choose An Option That Best Describes Your Problem. Cuts an angle in half to.

How To Find Orthocenter Of A Triangle 4 Easy Steps Video

The point when all three perpendicular bisectors meet.

. Find the slopes of the altitudes for those two sides. It does so whenever the triangle is obtuse. In the next section we will discuss the orthocenter centroid circumcenter and incenter of a triangle.

Was this answer helpful. The orthocenter is the point where the 3 altitudes of the triangle intersect. For an acute angle triangle the orthocenter lies inside the triangle.

There are therefore three altitudes in a triangle. If VX9 find VY and YX. Find the equations of two line segments forming sides of the triangle.

On the other hand the orthocenter intersection of thealtitudes can. The point where the perpendicular bisectors of the three sides of. Which best describes the orthocenter of a triangle.

The orthocenter of a triangle is defined as. You can find where two altitudes of a triangle intersect using these four steps. The point where the three medians of the triangle intersect.

The orhocenter of a triangle is the point where the altitudes from the 3 vertices of the triangle meet. The point when all three altitudes meet ON EULERS LINE Perpandicular line through a point. A point of concurrency is the intersection of 3 or more lines rays segments or planes.

Let O0 0 A 4 0 and B3 4 be the vertices of the triangle. Solve the corresponding x x and y y values giving you. The orthocenter of a triangle is the intersection of the triangles three altitudes.

The point of intersection of the three heights of a triangle. An altitude is a line which passes through a vertex of the triangle and is perpendicular to the opposite side. The centroid of a triangle is that balancing point created by the intersection of the three medians.

The Orthocentre Of A Triangle With Vertices 0 0 3 4 And 4 0 Is. Orthocenter of a Triangle. The orthocenter is the point of concurrency of the altitudes in a triangle.

The orthocenter is just one point of concurrency in a triangle. Therefore slope of OP ie OHslope of BA -1. 4 none of these.

For the obtuse angle triangle the orthocenter lies outside the triangle. The point where the three altitudes of the triangle intersect B. Definition of the Orthocenter of a Triangle.

The point where the three medians of the triangle intersect O D. 4 rows It turns out that all three altitudes always intersect at the same point - the so-called. The orthocenter is one of the triangles points of concurrency formed by the intersection of the triangle s 3 altitudes.

The incenter of a triangle is the point at which the 3 medianslines from the vertex to the middle of the side opposite thevertex of the triangle intersect. GRAPH the triangle will the orthocenter be inside outside or on the triangle. Use the slopes and the opposite vertices to find the equations of the two altitudes.

The incenter of a triangle is the point at which the 3 medians lines from the vertex to the middle of the side opposite the vertex of the. For the obtuse angle triangle the orthocenter lies outside the triangle. The orthocenter H of a triangle is the point of.

The point where the three angle bisectors of the triangle intersect O C. Sketch the altitudes from each vertex this will help you visualize where the orhocenter is Give the intersection of the altitude and base a point 3. The orthocenter of a triangle is the point where the perpendicular drawn from the vertices to the opposite sides of the triangle intersect each other.

Find the slopes of 2 of the sides of the triangle 4. Equal distant from each side of the triangle NOT ON EULERS LINE Makes a circle in the triangle. Let H be the orthocenter of OAB.

The orthocenter is the point where all three altitudes of the triangle intersect. It has several important properties and relations with other parts of the triangle including its circumcenter incenter area and more. Florianmanteyw and 21 more users found this answer helpful.

A segment drawn through the midpoint of a side and at a right angle to the side. The orthocenter of a triangle is the point where the perpendicular drawn from the vertices to the opposite sides of the triangle intersect each other. The point where the three altitudes of the triangle intersect.

These three altitudes are always concurrent. For an acute angle triangle the orthocenter lies inside the triangle. The point where all the three altitudes of the triangle meet or intersect each other.

Per its definition the incenter cannot ever fall outside thetriangle. An altitude is the line drawn form one of the vertices of the triangle at right angles to the line opposite the vertex. The point where the perpendicular bisectors of the three sides of the triangle intersect.

In other the three altitudes all must intersect at a single point and we call this point the orthocenter of the triangle. In the case of the above triangle we can denote it as.

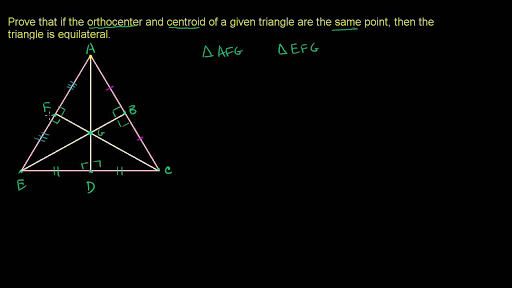
Common Orthocenter And Centroid Video Khan Academy

Triangle Centers

How To Find Orthocenter Of A Triangle 4 Easy Steps Video

How To Find Orthocenter Of A Triangle 4 Easy Steps Video